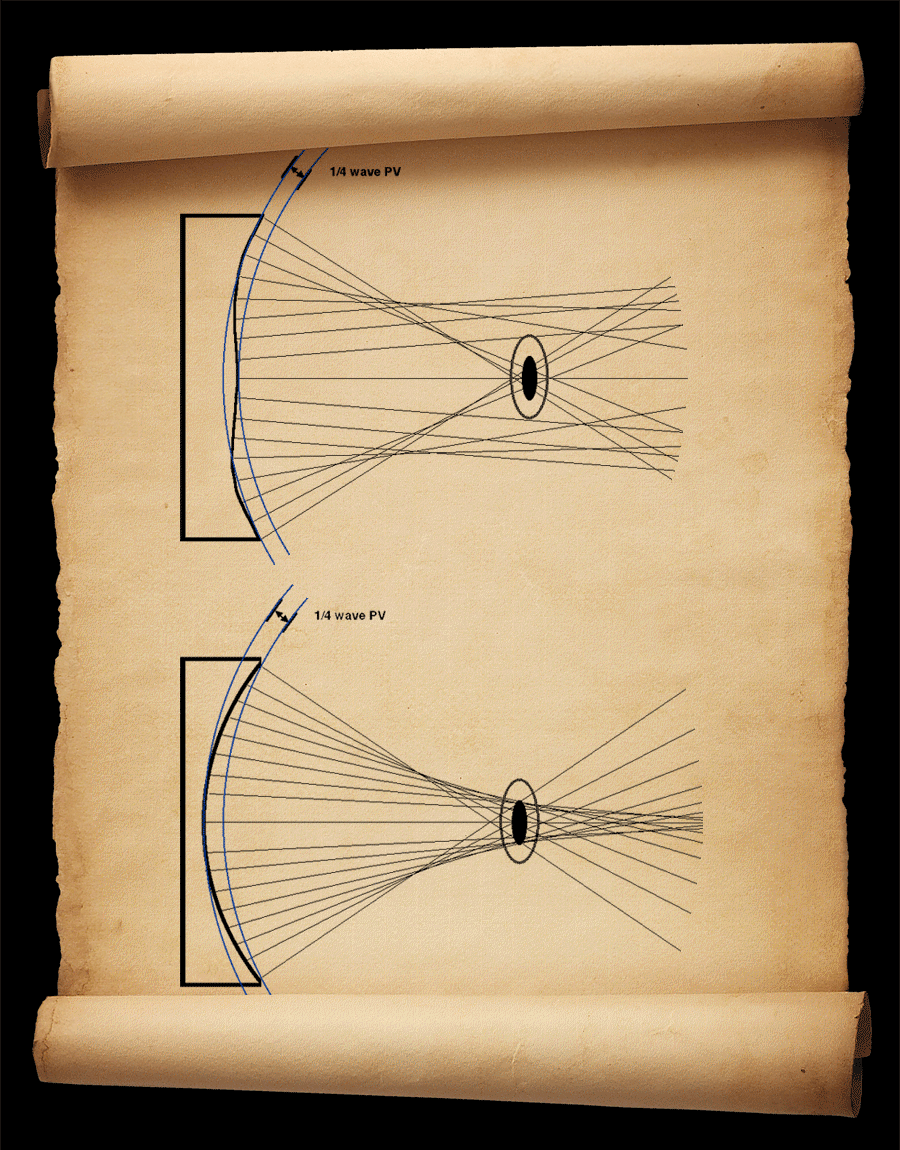
Criterion #2

The large scale surface will be smooth and regular for a given focal ratio, with transverse aberration not exceeding the diffraction disk at the focal plane.

In the book Star Testing Astronomical Telescopes, (the definitive work on the subject of total telescope system performance), author Dick Suiter in the first chapter addresses our second criterion. On page 7, in the section titled "Measures of Optical Quality" is a diagram of two primary mirrors, demonstrating the difference of surface slopes. Both are 1/4-wave mirrors, but because of the smoothness (or roughness) of the corresponding large scale surfaces, both demonstrate a different result at the focal plane.

Below we have two diagrams similar to those in Suiter's book, showing this concept. Both examples are 1/4 wave mirrors, having all surface height deviations fit within a wavefront shell of two spheres, both of which have a common center at focus, and are separated by 1/4 wave. However within that tolerance envelope are two different kinds of surfaces. The difference between the two optics is not their wavefronts, but their surface slopes as measured at the focal plane.

The top diagram has slope errors which when ray-traced, direct the light a great distance outside the airy disk, or diffraction disk. The optic in the second diagram has the same wave rating, but has no light directed outside the diffraction disk. The black circle in each drawing represents the airy disk, and the ring around it the first diffraction ring. The size of the diffraction disk in an F/4.5 system for example is .00012 inches (one-point-two ten-thousandths of an inch). (Drawings are for conceptual demonstration only, and are not to scale).

The key concept here is “slopes". At Zambuto Optical we actually measure for this criterion. We are keenly interested in controlling the slopes and how they affect the focal plane, because it is a much more sensitive criterion than controlling the mirror’s surface height, given a particular method of measurement. This notion dates back to 1935 as addressed by Danjon and Couder. They put forward two criteria for optical quality, the second of which was the Raleigh Criterion, where 1/4 wave is obeyed over the entire optic, and over most of the aperture it is appreciably less. However their first criterion is significantly more stringent- “Over the greatest part of the aperture the wavefront has a mild slope and does not divert rays outside the diffraction disk". This second criterion actually produces essentially a 1/7 wavefront standard in and of itself.

For optics of F/4.5 and longer, we target 3/4 of this diffraction disk tolerance as our standard. And for F/5 and longer we target 1/2 the diffraction disk. These slope tolerances are much more difficult to achieve than wavefront height deviation alone, because there are many possible quality levels within a given wavefront tolerance, as seen conceptually, in the images above. Example, it is actually possible to optically fabricate and measure an optic whose spherical aberration does not exceed 1/10 wavefront, but whose slopes actually exceed the diffraction disk at the focal plane.

This standard is called the Millies-Lacroix Tolerance. We use it because it insures optimal large scale surface smoothness, and so is a major component in our seven criteria for world class performance. We're going to visit this concept again at a smaller scale in criterion #4, where we will find something rather surprising about test methods.